

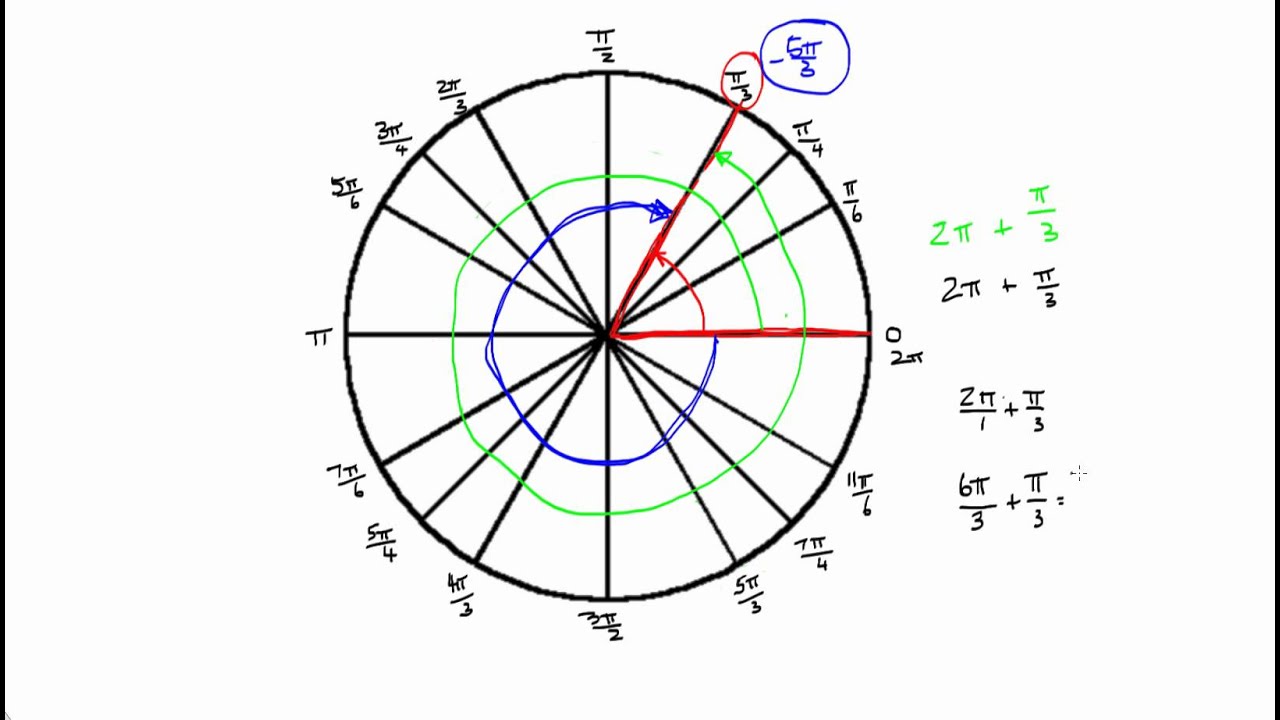
For reasons you'll learn later, mathematicians like to work with the "unit" circle, being the circle with r = 1. You know that the circumference C of a circle with radius r is given by C = 2π r. Why is the value for one revolution in radians the irrational value 2π? Because this value makes the math work out right. The 360° for one revolution ("once around") is messy enough. Something similar is going on here (which will make more sense as you progress further into calculus, etc). Yes, " 83%" has a clear meaning, but to do mathematical computations, you first must convert to the equivalent decimal form, 0.83. This is somewhat similar to the difference between decimals and percentages. Why do we have to learn radians, when we already have perfectly good degrees? Because degrees, technically speaking, are not actually numbers, and we can only do math with numbers.
(Click "Tap to View Steps" to be taken directly to the Mathway site for a paid upgrade.)

Please accept "preferences" cookies in order to enable this widget. Just as "hours" can be expressed as decimal hours or else as "hours - minutes - seconds", so also "degrees" can be expressed as decimal degrees or else as "degrees - minutes - seconds", denoted as "DMS". These units, just as for "hours", are called "minutes" and "seconds". But just as "1.75" hours can be expressed as " 1 hour and 45 minutes", so also "degrees" can be expressed in terms of smaller units. When you work with degrees, you'll almost always be working with decimal degrees that is, with degrees expressed as decimal numbers such as 43.1025°. When you're doing graphs and drawings involving measured angles, you'll be starting with 0° being "east" (it'll actually be the x-axis), and you'll rotate anti-clockwise. Whatever convention your book uses should be specifically defined in the book ask your instructor, if it isn't otherwise clear.Īnd yes, this way of measuring direction (namely, starting at north and moving clockwise) is different from how you'll be measuring angles. These mean " 36 degrees west of north" and " 27 degrees east of south", respectively. Another way of giving directions using degress is of the form N36°W or S27☎. Note: When directions are given in terms of degrees, the direction is (usually) found by starting at "north", being 0°, and moving clockwise by the number of degrees given.


 0 kommentar(er)
0 kommentar(er)
